
Introduction to Tessellations (Tilings)
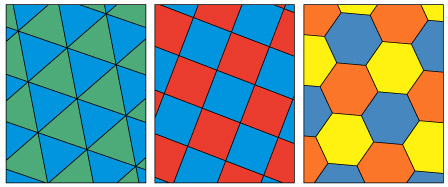
Regular tessellations
A regular tessellation is one made up of regular polygons which are all of the same type, and for which all vertexes are of the same type. (A vertex is a point at which three or more tiles meet.) There are only three regular polygons that tessellate in this fashion: equilateral triangles, squares, and regular hexagons.
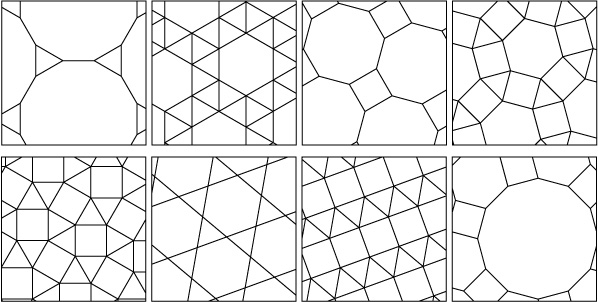
Semi-regular tessellations
A semi-regular tessellation is one made up of two different types of regular polygons, and for which all vertexes are of the same type. There are eight semi-regular tessellations. The three regular and eight semi-regular tessellations are collectively known as the Archimedean tessellations.
Tessellations around us
Tessellations are widely used in human design. Examples include floor tilings, brick walls, wallpaper patterns, textile patterns, and stained glass windows. Basically, anytime a surface needs to be covered with units that neither overlap nor leave gaps, tessellations come into play.
Repeating and non-repeating tessellations
Tessellations do not have to be repeating, or periodic. In fact, there is a famous family of tessellations based on two tiles known as "Penrose" tiles. These are named after their inventor, English mathematician and theoretical physicist Sir Roger Penrose. When assembled according to a simple set of matching rules, an infinite number of distinct tessellations can be formed by Penrose tiles, and none of them are repeating! These tiles have a number of other interesting properties, many of them related to the Golden Ratio.
Click here to browse products related to tessellations.
Math + Art topics
mathartfun.com home
© 2000-2015 Tessellations



