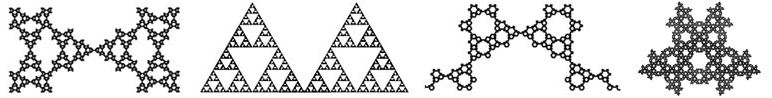
Gasket Fractals

These fractals are formed by removing one or more tiles from a subdivided self-replicating tile and then iterating. A self-replicating tile, or "reptile", is a tile that can be tiled with smaller copies of itself. The two rectangles near the bottom of the page are not reptiles but still allow the construction of gasket fractals. Click on the image buttons to see gasket fractals based on the tile shown.
Other than the Sierpinski Triangle and Sierpinski Carpet, I discovered all of these myself, though I subsequently found (not to my surprise) that several of them have been previously discovered by others. While I haven't made an exhaustive search to find what others have done, notes are made on the individual pages when there are earlier examples of which I'm aware.
This sort of fractal, a type of iterated function system, is treated more mathematically by others. My focus on these objects is more as graphic objects, and the examples I've included here were largely chosen because they appealed to my sense of esthetics.












































All images copyright Robert Fathauer
Fractal Diversions home
Fractal Tiling
 Fractal Knots
Fractal Knots
 Gasket Fractals
Gasket Fractals
 Fractal Trees
Fractal Trees
 Hyperbolic & Folded Fractals
Hyperbolic & Folded Fractals
 Polyhedra
Polyhedra
 Papers
Papers